Fractions are a fascinating topic and a bridge to so many different areas of mathematics. Recently I’ve been thinking about how students develop an understanding of fractions and how to support them in building their curiosity and enjoyment of what they’re learning. Here are four powerful ideas for helping students build skills and confidence in this topic:
1. Numbers on a Number Line.

Present students with a number line (e.g. from 0 to 1) and different fractions (e.g. 1/4, 1/2, 1/8) for them to place. Ask for justifications for where they place the fractions. Repeat the activity regularly using different number lines (e.g. 0 to 2) and fractions (e.g. 3/3, 5/3, 1/6).
This helps students to: describe fractions accurately (e.g. "three quarters" not "three over four"); and understand that the numerator and denominator are part of the same number, rather than being distinct values.
2. Which shows one third?
Present students with a range of diagrams. Ask: Which show one third? Can you draw other shapes that show one third? How do you know? Discuss what makes one third. Repeat the activity using different target fractions.
This helps students to: understand that the value of the numerator (e.g. 2 in 2/3) refers to equal sized parts.
3. Smaller or larger?
Using a fraction wall, ask students to form fractions and compare their lengths. Which is smaller: 1/3 or 1/5? 1/3 or 4/5? Can you find some other fractions that are smaller than 1/3? Can you find fractions that are larger than 1/3? How do you know? Repeat the activity using different fractions (e.g. 1/4 vs 2/9, 1/4 vs 3/8).
This helps students to: not rely on misleading ‘rules’, e.g. 1/3 is smaller than 1/5, because 3 is less than 5; or 1/3 is greater than 4/5 because the smaller the denominator the bigger the fraction.
4. Making sense of operations.
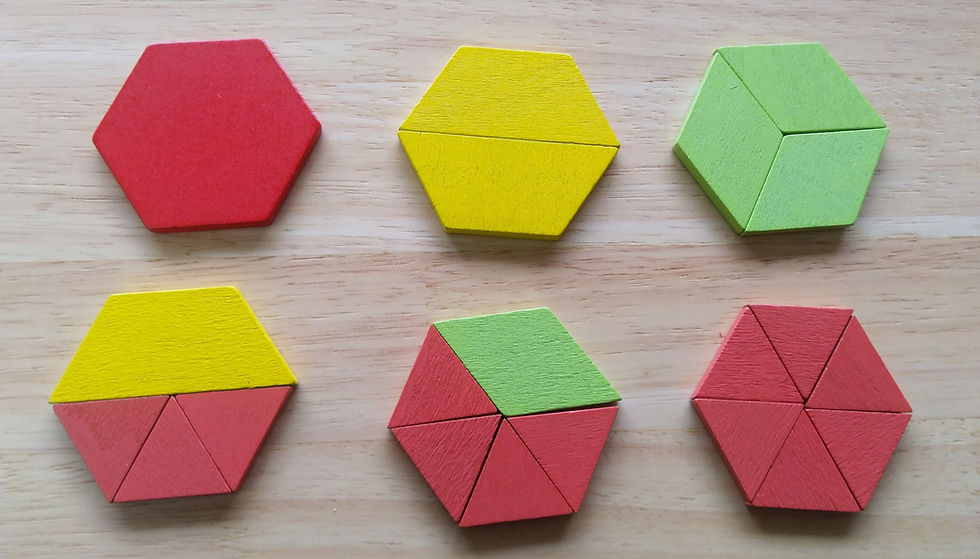
When introducing operations with fractions, emphasise estimation and how reasonable answers seem. Use visual and concrete models, like fraction walls, grids, counters, number lines and pattern blocks.
This helps students to: not mistakenly apply the methods of operating with whole numbers, to operating with fractions, e.g. to know that 1/2 + 1/2 ≠ 2/4.
Find Out More
For more information on supporting students, check out Maths Teacher Circles virtual workshops on using engaging ideas for teaching maths meaningfully.
I also recommend these excellent resources:
Top Drawer Teachers [online resources] from the Australian Association of Mathematics Teachers
Elementary and Middle School Mathematics: Teaching Developmentally [book] by Van de Walle, Karp and Bay-Williams
What is your experience with these ideas and tools? Do you have other tried and tested advice that is helpful for teaching fractions?


Comments